mathworld.wolfram.com/HypercubeLinePicking.html

Preview meta tags from the mathworld.wolfram.com website.

Linked Hostnames

6

Thumbnail

Search Engine Appearance

Google

https://mathworld.wolfram.com/HypercubeLinePicking.html

Hypercube Line Picking -- from Wolfram MathWorld

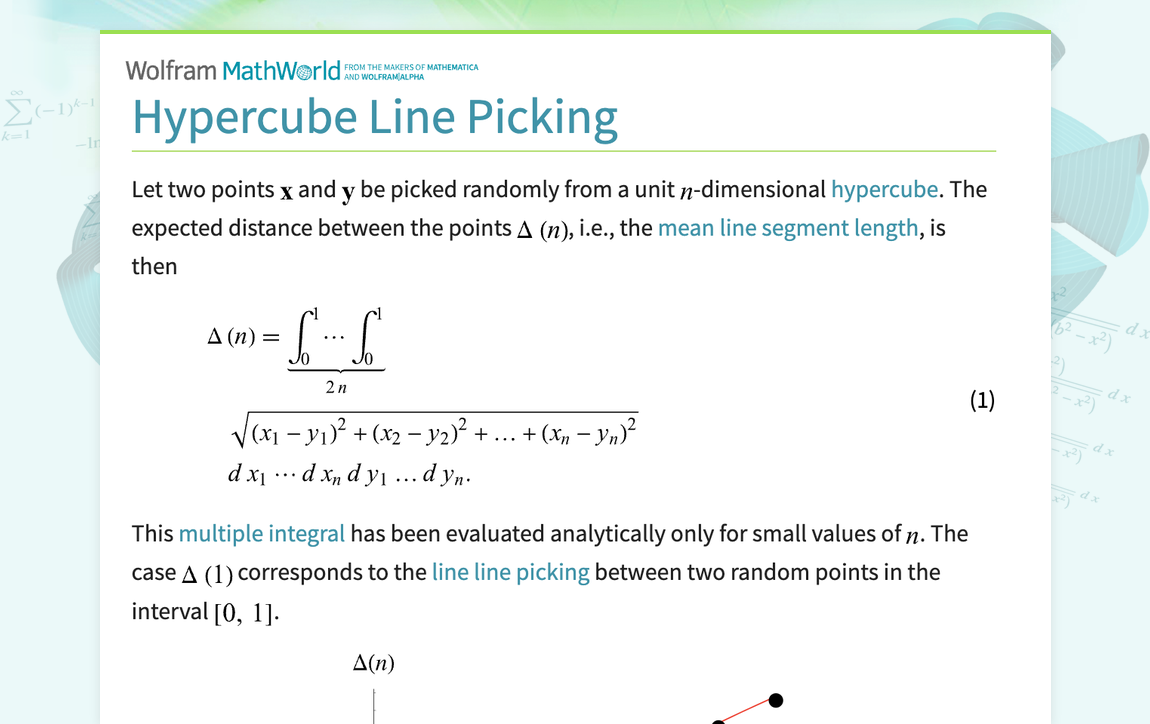
Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...



Bing

Hypercube Line Picking -- from Wolfram MathWorld

https://mathworld.wolfram.com/HypercubeLinePicking.html

Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...



DuckDuckGo

https://mathworld.wolfram.com/HypercubeLinePicking.html

Hypercube Line Picking -- from Wolfram MathWorld

Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...

  • General Meta Tags

    36
    • title
      Hypercube Line Picking -- from Wolfram MathWorld
    • DC.Title
      Hypercube Line Picking
    • DC.Creator
      Weisstein, Eric W.
    • DC.Description
      Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...
    • description
      Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...

  • Open Graph Meta Tags

    5
    • og:image
      https://mathworld.wolfram.com/images/socialmedia/share/ogimage_HypercubeLinePicking.png
    • og:url
      https://mathworld.wolfram.com/HypercubeLinePicking.html
    • og:type
      website
    • og:title
      Hypercube Line Picking -- from Wolfram MathWorld
    • og:description
      Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...

  • Twitter Meta Tags

    5
    • twitter:card
      summary_large_image
    • twitter:site
      @WolframResearch
    • twitter:title
      Hypercube Line Picking -- from Wolfram MathWorld
    • twitter:description
      Let two points x and y be picked randomly from a unit n-dimensional hypercube. The expected distance between the points Delta(n), i.e., the mean line segment length, is then (1) This multiple integral has been evaluated analytically only for small values of n. The case Delta(1) corresponds to the line line picking between two random points in the interval [0,1]. The first few values for Delta(n) are given in the following table. n OEIS Delta(n) 1 -- 0.3333333333... 2 A091505...
    • twitter:image:src
      https://mathworld.wolfram.com/images/socialmedia/share/ogimage_HypercubeLinePicking.png

  • Link Tags

    4
    • canonical
      https://mathworld.wolfram.com/HypercubeLinePicking.html
    • preload
      //www.wolframcdn.com/fonts/source-sans-pro/1.0/global.css
    • stylesheet
      /css/styles.css
    • stylesheet
      /common/js/c2c/1.0/WolframC2CGui.css.en

Links

74